Median Data || Describing Data: Summary Measures || Bcis Notes

Median Data

The variable values dividing the total set of observations into two equal parts of the data is called the median data.

For a data set, it may be thought of as the “middle” value.

For example, in the data set [1,3,3,6,7,8,9], the median is 6, the fourth largest, and also the fourth smallest, number in the sample.

For Individual Series

1. First, we change the data in ascending or descending order of their magnitude.
2. If the number of observations is odd, then the middle value gives the median among all the data.
3. Again, if the number of observations is even then there will be two middle values, the average of two middle values gives the median.
4. The formula for computing the median in case of individual series is given by

where,

n=Number of Observations

For discrete series,

1. Arrange the data in ascending order of their magnitude.
2. From the cumulation frequency table.
3. Find the value of  .
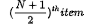4. See the cumulation frequency table equal to or just greater than .
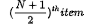5. Note the corresponding value of x which gives the value of the median.

where,

N=Number of Observations

For Continuous Series,

1. Prepare a c.f. table.
2. Find
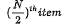
3. See if the value in c.f. the table is equal to or greater than 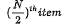, note the class.
4. The corresponding class contains the value of the median and is called the median class.
5. The following formula is used in finding the median:

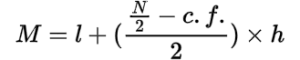
where

l=lower limit of the median class

f=frequency of the median class

c.f.=c.f. of preceding median class

h=size of the median class

 

You may also like Arithmetic Mean